Specific Heat of Liquid "X"

The specific heat of Liquid "X" is to be measured. 250 grams of the liquid are placed in a test apparatus. An instrument inserted into the liquid functions as a heater, stirrer, and thermometer (H-S-T). The power to the heater-stirrer is 15 watts. When it is turned on, the temperature rises for a while then becomes constant. When the power is shut off the thermometer indicates that the temperature decreases at the rate, 1.2°C/min. A specification of the test apparatus is that 50 Joules are required to change its temperature 1°C.

Calculate the specific heat of Liquid "X".

Tutorial: This system of this has two components. The event, a rate type, has a subtle aspect. Enthalpy is expressed in two manners. Consequently this solution will be conducted as a tutorial.

Mass Equation: Since every system has mass, mass is a convenient first consideration. In this case the system is the liquid (mass = 250 grams) and the instrument for which the mass is unknown. (Part of this solution is to explain how the instrument mass is included implicitly.) So there are two mass-entities: the fluid and the H-S-T instrument; both remain constant for the event.

Given no further detail about the test apparatus, we must make some assumptions. We assume the H-S-T to be completely submerged in liquid "X". Thus, by this definition, there will be no heat associated with it. The instrument will experience energy change by electric work and it will thermally equilibrate with the liquid. Further we assume the boundary of the liquid, all but its surface, to be ideally insulated (///////).

Energy Equation: We start with a general energy equation. Kinetic energy of the liquid will be rotational and small and its potential energy is constant. Both energies are constant for the instrument.

(1)(1)

The event involves the liquid and the instrument. Thus there are two "rates of internal energy change" and two "work rates" (right-of-equality). We leave the "heat rates" represented as a sum.

(2)(2)

We identify both substances as being "simple compressible" meaning that the only manner of energy change by work is "change of volume." In thermo parlance, each has the "pdV" work mode, only. We represent that the fluid is stirred by the term "work-rate of friction." Rewriting the equation yields:

(3)(3)

Process: The next idea to apply is named "process." Very many events of thermodynamics occur in the ambient atmosphere and in time periods over which the assumption "constant pressure" is realistic. This measurement of specific heat occurs at constant the constant pressure, 1 atmosphere.

When pressure is constant, work integrals (for the liquid and instrument) are readily rearranged as below:

(4)(4)

Once the work integrals (simple compressible) are changed to "constant pressure" forms, it is convenient to move them left-of-equality, then group each of them with their respective internal energies.

(5)(5)

Both terms, left-of-equality can be written in the form, d[U + pV]/dt. This group of terms, U + pV, appear so often in thermodynamics that it is given its own name and symbol: enthalpy with the symbol H.

(6)(6)

For the "liquid" we express the enthalpy as its mass times its specific enthalpy, i.e., H = mh. And lastly, we show the "dependence" of enthalpy (stated by classical thermodynamics) explicitly as being of temperature and pressure. So our variable change for the liquid is H = mh(T,p). Since mass is constant we can write:

(7)(7)

Enthalpy is not directly measurable. Its change for an event is made quantitative in terms of the changes of its properties, p and T. Since enthalpy is a function of two variables, partial differentiation is required to express its differential. Two terms result, the second of which equals zero for constant pressure.

(8)(7)

The partial derivative of the first term is measurable. It is called the specific heat at constant pressure of the fluid. The units of the number are E/mθ. Now we represent the energy change of the liquid as its mass (0.25 kg) times its specific heat times its time-rate change of temperature. The term right-of-equality in Equation (8) is used to replace the first term (left-of-equality). Also, the power of the instrument is -15J/s. We include this fact is to write our energy equation as:

(9)(9)

It makes sense to state the change of temperature of a liquid in terms of a unit mass of that liquid and that is the way it is done for liquids (first term left above equation). However the change of energy of an instrument sometimes occurs. It makes little sense to cast that change in terms of a "unit mass" of the instrument. Rather, a specific heat for the instrument "in its entirety" is used. The units are (E/θ). The term, so expressed, is second left-of-equality, below:

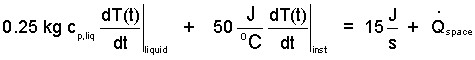
(10)()

Event: Equation (10) relates the energy interactions of the liquid and instrument in a differential manner. The equation tells how "instantaneous changes" of the the system materials occur relative to electric power work and heating to the surroundings. The above equation describes our system changes as they happen leading to the commencement of our "specific heat measurement event."

Once the H-S-T (Heater-Stirrer-Thermometer) is turned on (at a constant 15 watts) the temperature (of the liquid and instrument) indicated by the thermometer will increase steadily then attain a constant, maximum value temperature. Upon this condition, the liquid temperature and instrument temperature will no longer change. Energy Equation (11) expresses this condition: D317_e09

(11) (11)

Equation (11) states that 15 Joules of heat pass from the system to surroundings immediately prior to our measurement event.

(12)(12)

Measurement Process: Measurement of the liquid specific heat is accomplished by observation of the cooling of the system from its beginning temperature, the condition expressed by Equation (12). A fundamental assumption here is, upon shut-off of the power, the "cooling rate" (being equal to the electric power), at the moment the power is turned off, will be constant for at least a few moments. For those moments this is true, the energy equation is:

(13)(13)

Equation (13) contains the temperature of the liquid and of the instrument. For the equilibrium condition attained (Equation (11)), those temperatures are the same. We also assume them to be equal for the moments just after the electric power is terminated.

(14)(14)

Finally, to obtain the average specific heat (over the two degree temperature range) we solve Equation (14). The procedure is to separate variables then integrate. The initial temperature is set to be T* which occurs at the instant the electric power is shut off, t*.

(15)()

Finishing the details, we obtain:

(16)(16)