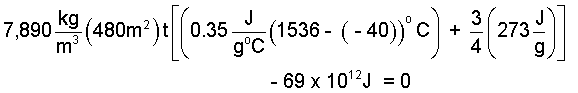Space Shuttle Re-entry

The shuttle has a mass 2.7 million kilograms. When it returns from mission, its re-entry commences with a speed near 7000 meters per second and at an altitude of about 125 kilometers. The shuttle must descend and arrive at a runway with a landing speed of 160 m/s. This happens quickly - in about 30 minutes. Thus the immense kinetic and potential energy of the orbiting craft must be dissipated somehow and somewhere during descent through the atmosphere.

Initially the shuttle has large kinetic and potential energies which must be dissipated to the atmosphere. The mechanism for it to slow and descend is aerodynamic drag which causes the shuttle to become very hot. This heat must be dissipated; convective heat transfer helps it stay cool, radiant heat transfer is less. The process of reentry is complicated.

So, at our level, can anything be calculated regarding a re-entry event? Are there any calculations, though admittedly imaginative, that might "give a feel" or a "sense of magnitude" to the frictional equilibration attendant to re-entry? Can we reasonably portray just how great an amount of energy must be dissipated with each re-entry. Only in a very approximate way. To begin we write and simplify the energy equation for the event.

Mass Analysis: Supposing none of the shuttle melts, the mass will be constant.

Energy Analysis Perform an energy analysis of a reentry.

♦ It is clear that air, the surrounding atmosphere, is involved. Therefore we take the shuttle and a big piece of air (in the flight path) as our system. As will be shown, a precise description of the air-part of the system is not needed. We use an energy equation with the notation, Σ meaning a "discrete sum" over the distinct parts of the system. In this case, the sum includes the shuttle and the air. The equation:

(1) 1

In beginning-level thermodynamics heat is the easiest term to specify. It is either i) given in the problem statement, or ii) all other terms are known so heat is "solved for" or iii) it is zero. Heat is zero with assumed "perfect insulation" or for events that proceed to quickly for there to be heat. The re-entry is not prompt.

In the above equation ΣQ represents all energy interactions of the shuttle and atmosphere with surroundings (i.e., with deep space or with Earth) that occur because of a temperature difference. The shuttle is contained by the atmosphere - there is no heat there because there is no system boundary there. Does air at the Earth surface (or the top of the atmosphere) become hot that there is heat to the ground? Nonsense. There might be a small amount of heat radiated to space from the glowing shuttle but we assume that is small. That heat is zero is a reasonable (and necessary) approximation. We drop heat and expand the "energy side" of the equation.

(2) 2

We next address the work of the event. It is logical to suppose there is no work nor heat with the vast system we have chosen. What about compression work? The vast atmosphere has zero pressure above it but it probably expands. We choose not to discard work but rather to lump the compression work with internal energy to be represented as enthalpies.

(3) 3

Next we rearrange and apply the numbers:

(4) 4

This large amount of energy, initially possessed by the shuttle, agrees with what is known. When the shuttle re-enters, it dumps a great deal of energy to the atmosphere but in doing so a large amount comes to reside in the shuttle itself. This aspect of shuttle mechanical energy dissipation is called frictional heating of reentry.. But from our system perspective, the event is one of equilibration wherein the shuttle mechanical energy diminishes and the thermal energy of the atmosphere and shuttle increase. Shuttles are fitted with "heat shields" to protect them upon re-entry. We wonder just how big of a problem that much energy might be.

IRON:
ρ_s (g/cm³)	c (J/gK)	T_mp (°C)	h_sf (J/g)
7.89	0.35	1536	273

Steel, Non-Melting Shuttle Shield: It is proposed, to act as a "heat shield," to attach a uniformly thick plate of steel to the bottom of the shuttle. As a first design, we assume the worst case, that the shield must absorb the entire energy change of re-entry distributed evenly in the shield and that none of the steel melts. Calculate the thickness of shield required.

♦ In accord with our assumptions the energy change of the atmosphere will be zero. The steel (iron) of the shield will experience a uniform energy increase to, but not to exceed, its melting temperature. We write the energy change of the steel in terms of its mass, average specific heat (as solid) and its temperature change for the event.

(5) 5

The mass of the slab of iron of the shield equals iron density times volume, or density times the area times thickness of the shield. A reasonable assumption for the initial temperature of the shuttle steel, deep in space, is -40°C. The steel can get as hot as its melting temperature which from the table is 1536°C.

(6) 6

I checked the numbers. That is a lot of steel. To attach a slab of steel 33 meters thick to the bottom of the shuttle is absurd. Sometimes a simple thermodynamic calculation will tell one just how silly is an idea is. But to give steel a second chance; suppose we design to let some of the steel melt and blow away.

Steel Shield with 75% Melting: Supposing 3/4 of a steel shield might melt and blow away into the atmosphere, what initial thickness of shield would be needed?

♦ Ablation is the processes whereby steel of the shield, becomes progressively hotter with motion then melts and is blown away to the atmosphere. In that process some energy goes to the surrounding air and some to the shuttle. The worst case is if ALL energy goes to the shield.

(7) 7

A real shield will not ablate uniformly but in this calculation we assume ours does. The event of the steel shield is an increase of energy (temperature) of "all of the steel" from -40°C to melting at 1536°C, and thereafter to receive further energy until 3/4 of its thickness is caused to ablate (melt and blow away) into the atmosphere. What original thickness of shield do we need?

(8) 8

The energy equation (in accord with our assumptions) states the energy change of the shield as two components. Initially energy arriving to the shield disperses warming the steel uniformly to its melting temperature. Thereafter, by our further assumption, more energy arrives and the steel commences to melt and once liquid, be blowm away by the motion (to ablate). The second term accounts for ablation principally by its component, the "melting" energy or h_sf of iron (273 J/g). Putting the numbers in:

(9) 9

This is now a single equation with only one unknown, the shield thickness t. We solve it to determine the thickness: t = 24.3 meters. Phew! (Much steel. Re-Check the numbers!) Our assumptions were "worst scenario" but obviously, steel is not the material for this job as the US Space agency knows. Ceramic materials are the best shuttle heat shields.