| THERMO Spoken Here! ~ J. Pohl © | TOC NEXT ~ 196 |
Pressure Cooker
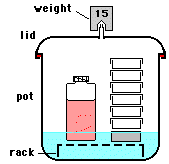 Pressure cooking is largely an industrial process but many restuarant kitchens also pressure cook (PC). Thorough analysis of a pressure cooker events are tedious because their events are transient and open-system.
Pressure cooking is largely an industrial process but many restuarant kitchens also pressure cook (PC). Thorough analysis of a pressure cooker events are tedious because their events are transient and open-system.
The discussion here was motivated by a text example in Engineering Thermodynamics by Cengel and Boles. The figure shown is from that text. The problem statement was:
A pressure cooker that has attained its operating pressure of 175kPa has expelled all air. It contains 1 kilogram of water as liquid and vapor. The volume of the cooker is 0.006 cubic meters. Suppose heat to the cooker occurs at 500J/s for 30 minutes. Calculate the final masses of liquid and vapor water in the cooker.
Below is an alternate solution of this example. Before we begin, it is helpful to realize that in thermodynamic analysis there is no specific, easiest way of solution. This is to say there is no specific "place" to begin and steps need not be done in any order. Analysis involves mass, state (initial and final), energy, property equations, heat, work, characteristics of the event and solution techniques. There are many paths to the solution of any thermo problem. Below, for this pressure-cooker, one path to solution is demonstrated one way.
EVENT: The pressure cooker (water within the interior volume as system) involves changes of mass and energy. The mass equation is easier than the energy equation. Therefore we develop the mass equation first. The generic mass equation (suited for all systems and all events) is:

| (1)
Generic mass equation. |
This is a first order differential equation with time as the independent variable. The event of this cooker is "start-stop" in nature. We identify the "start" time as t = 0+. The event is stated to end later, after 30 minutes; t = 30 min. So we integrate between those limits.

| (2)
Integration between limits of event time are applied. |
The term left of equality integrates immediately!

| (3) 3 |
Inspection of the integral right-of-equality reveals that it can be integrated provided the time dependence of its integrand, the rate of mass leaving the cooker can be specified over the 30 minutes of the event. That is, does more mass leave initially and less later or what? Since this time-dependence is not known, must our analysis end here? With any analytic situation such as this, an engineer makes every effort to get some manner of answer. One technique applied to integrals the integrands of which are unknown is apply the Mean Value Theorem of calculus. The steps are as follows:
Replace the time dependent integrand, [m-dotout(t)] with its "average," [m-dotout,avg], then integrate. So what we did is replace a time dependent term we did not know with another term we don't know.
- The function we do not know is the mass out [or m-dotout(t)], over the time of 30 minutes.
In the above equation (3) the product "m-dotout,avg times Δ t" is an amount of mass. What is left of the equality is an increment of mass, Δm = m2- m1. But physically "m-dotout,avg" times "Δt" is not an increment. Thus "m-dotout,avg times Δt" is written as a lower case " δ " type of increment.
These steps to arrive at the above mass equation are used for many problems. For this Pressure Cooker, the authors have made it clear that the initial state of the water is two-phase. It is not known whether the second (final state after 30 minutes of heat) will be two phase or all vapor. Consequently, assume: the second state is two phase, then extend the mass equation:
| [mvapor,2 + mliquid,2] - [mvapor,1 + mliquid,1] = δmass out | (5)
5 |
| Properties of Water p = 175kPa |
| Tsat = 116°C |
| vf = 0.001 m3/kg |
| vg = 1.00 m3/kg |
| uf = 487 kJ/kg |
| ug = 2038 kJ/kg |
| hf = 487 kJ/kg |
| hg = 2701 kJ/kg |
In this case the initial state is known to be two phase water at 175 kPa with a volume of 0.006 m3 and a mass of 1.0 kilograms. Immediately we have:
| mvapor,1 + mliquid,1 = 1.0 kg | (6) 6 |
|
mvapor,1vvapor + mliquid,1vg = 0.006m3 or
| (7) 7 |
| () |
mvapor,1 (0.001m3/kg) + mliquid,1 (1.00m3/kg) = 0.006 m3
So having done all we can with the mass equation we see we have three equations with five unknowns. The equations are:
(1) Initial mass of the system:
| mvapor,1 + mliquid,1 = 1.0 kg | (7) 7 |
(2) Volume of the system:
| mvapor,1 (0.001 m3/kg) + mliquid,1 (1.00m3/kg) = 0.006m 3 | (8) 8 |
(3) Mass Equation for the Event:
| [mvapor,2 + mliquid,2] - [mvapor,1 + mliquid,1] = δmass out | (9)
Reduced mass equation. |
The unknown terms are:
| mvapor,1, mliquid,1, mvapor,2, mliquid,2 and δmass out | (10) |
Next we address the energy equation.
 |
(11) |
For the pressure cooker...
(i) The system is only water so drop the 1st and 2nd summation signs (Σ).
(ii) The "in" terms do not apply.
(iii) Constant volume cooker has no "dV" hence no "pdV" work."
(iv) Kinetic and potential energy changes will be negligible.
(v) Assume there is no heat to the surrounding space. Drop the summation (Σ).
With these facts about the system, our equation reduces to this:
 | (12) |
So separate the variables and integrate the equation.
 |
(13) |
Hence, with energy, we have 4 equations and 5 unknowns. These equations are:
(1) Initial mass of the system:
mvapor,1 + mliquid,1 = 1.0 kg
(2) Volume of the system:
mvapor,1 (0.001 m3/kg) + mliquid,1 (1.00 m3/kg) = 0.006 m 3
(3) Mass Equation for the Event:
[mvapor,2 + mliquid,2] - [mvapor,1 + mliquid,1] = δmass out
(4) Energy Equation for the Event:
[mvapor,2uvapor,2 + mliquid,2uliquid,2] - [mvapor,1uvapor,1 + mliquid,1uliquid,1] = δmass outhmass out
The unknown terms are: mvapor,1, mliquid,1, mvapor,2, mliquid,2 and δmass out.
So we have 4 equations and 5 unknowns. This cannot be solved. We need as many independent equations as there are unknowns. What is the new equation we need? Answer: the second volume is also 0.006 m3.
Volume of the system in the second state:
(5) mvapor,2 (0.001 m3/kg) + mliquid,2 (1.00 m3/kg) = 0.006 m 3
The numbers are not important. There are sufficient equations to calculate the unknowns. We leave it here!