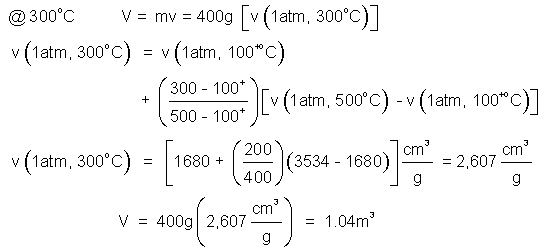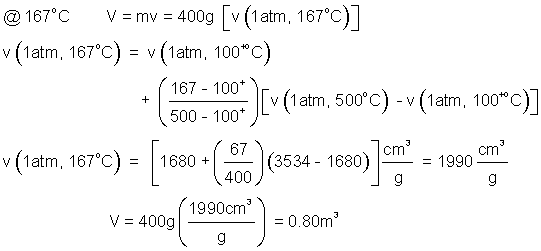| THERMO Spoken Here! ~ J. Pohl © | TOC NEXT ~ 1 |
WATER at 1 Atmosphere
The table presents selected thermodynamic properties of water at a pressure of One Atmosphere over the range of temperatures, -40°C to 500°C. This is the "solid-to-liquid" ~ melting phase change temperature and the "liquid-to-solid" ~ freezing temperature one atmpsphere. At 0°C, water is either solid (with solid properties) or liquid (with liquid properties). To tabulate both sets of properties at the same 0°, in the same column, temperature is awkward. Consequently we notate the solid to have the temperature 0-°C and the liquid to have the temperarure, 0+°C. Thus the temperatures, 0-°C, means "at 0°C" but solid and the temperature 0+°C means "at 0°C" but liquid. The same conventiion is used for 100°
The data of this table present states of water always at the pressure, 1 atmosphere over the temperature range, -40°C to 500°C).
| Water at One Atmosphere | |||||||
| p (Ts(p)) |
-40°C | 0-°C (solid~s) |
0+°C (liquid~f) |
100-°C (liquid-f) |
100+°C (gas-g) |
500°C | |
|
101.3 kPa (100°C) |
v ~ cm³/g u ~ J/g h ~ J/g |
1.08 -410 -411 |
vs = 1.09 us = -332 hs = -333 |
vf = 1.00 uf = 0.10 hf = 0.10 |
vf = 1.04 uf = 418 hf = 419 |
vg = 1680 ug = 2505 hg = 2675 |
3534 3130 3488 |
- - - - - - -
Problems below ask you to use the tabular data (water "at one atmosphere) to answer some questions regarding "state" and "events" of water occuring - at (the ambient pressure) 1 atmosphere.
1) 5000 grams of water exists at 1.0 atmosphere and 500°C. What is the volume of the water?
Solution: V = mv(1atm, 500°C) = 5000g (3534 cm3/g) = 17.7m3
2) Three grams of liquid water and 5000 cubic centimeters of gaseous water coexist in equilibrium at one atmosphere. What is the temperature? Calculate the enthalpy, H?
♦ Phases of water that coexist at one atmosphere have the temperature, 100°C. The enthalpy is:
H = mf hf + mg hg
H = mf hf + (Vg/vg) hg
H = 3g(419 J/g) + [5000 cm3/1680 cm 3/g](2675 J/g)
H = 9218 J
3) Determine the volume of 400grams of steam at 1.0 atmosphere and
a) 300°C. b) 167°C.
♦ 300°C is a nice number because it is midway between the table entries, 100+°C and 500°C. The fast answer is V = m{[v(500°C) + v(100+°C]/2}. Below we show all steps of the interpolation.