| THERMO Spoken Here! ~ J. Pohl © | TOC NEXT ~ 191 |
Citrus Concentrate

Sometimes orange juice is prepared from a "frozen-concentrate" product. The concentrate is shipped and sold with the instructions:
"Mix Concentrate with 3 Cans of Water."
Suppose immediately prior to mixing the concentrate is solid at -15°C and water from the tap is 20°C. What final temperature of the mixed juice is expected?
Before proceeding we consider some ideas about what the second temperature of this concentrate/water mixing event might be.
- We cannot calculate the precise second temperature of the concentrate/water mixture. Mixing requires time and with time there will be heat with the surroundings. Also a "mixing action," as by a stirring rod would deliver energy as frictional work to the mixture. So the second actual temperature is difficult to predict.
- We know with certainty that the highest second temperature of the orange juice will be room temperature, say 20°C. If mixing happens very slowly the OJ/water will attain room temperature.
- What might be the lowest possible temperatue of the OJ/water mixture? We can calculated "a lower" bound for the mixture temperature. This theoretically lowest possible second temperature would occur were the OJ and water perfectly and totally insulated and the mixing done without stirring or shaking.
a) We cannot know the precise temperature. However we know its limits.
Calculate the theoretical second temperature of the OJ once it is mixed. Assumes no heat with the surroundings, the solid and liquid are assumed "isolated" together within perfect insulation. Initially the orange concentrate, "one volume" of the can (with properties approximated as water), at - 15°C. The "three can volumes" of water added are at 20°C.
Solution: The mass equation for this event is quite simple, it direct and can be dealt with as we deal with the energy equation. The central form of the energy equation is the rate form.
 |
(1)(1-yes) |
There are two distinct masses: concentrate (conc) and the (tap) water. Thus we write the energy rates as two pieces (below - left of equality) and the sum of works is written as two "compression work" terms, (-∫pdv). Work of stirring and the sum of heats are set to zero.

| (2) |
(2-yes)
The energy (concentrate or tap water) is its internal energy and its kinetic energy (KE) and potential energy (PE), (known in terms of center of mass). We take the extrinsic energy changes to be negligibly small.

| (3) |
Thus our energy equation states "sum of internal energy changes equal the sum of compression works."

| (4) |
(4)
To solve this, some argue that the volume changes of the "work" terms of the concentrate and tap water are very small, whereupon:
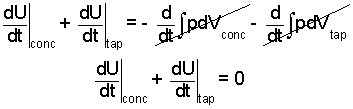
| (5) |
(5)
But our event occurs at the constant pressure. This permits us to reduce the compression work terms as follows:
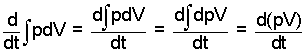
| (6) |
(6)
Combining the "∫pdv" result from Eqn-6 with the dU/dt's of Eqn-4, we obtain:

| (7) |
(4-yes)
Next, using the definition, H = U + pV, our energfy equation becomes (8a). We also write our mass equation as (8b):

|
(8a) |

| (8b) |
The procedure to change the rate form to the increment form follows steps (i) through (iv) (below).

| (9)(4-yes) |
The initial state of orange juice is a mix of "one can" of concentrate, with "3 cans" of tap water. The mass equation states simply that the mass of the system finally will equal the mass of the concentrate and water. Initially the concentrate is at -15°C and the three volumes of water are initially 20°C.

| (10)(4-yes) |

| (11)(4-yes) |

| (12)(4-yes) |
The water, finally, will have one temperature at one atmosphere of pressure. That temperature is unknown. The condition of phases is also not known. It might be all solid, at some temperature above 0°C, solid and liquid at 0°C or all liquid at some temperature. The subscripts of the mass equation (Eqn-3 - left-most term) indicate these possibilities.
One might wonder how large is the can, does the can size matter? The mass of a "can" of solid concentrate is essentially the same as the mass of that "can" filled with water. (The density of water as solid (ice) is about 4% less than as liquid). For this event the difference in liquid water mass per volume of can from solid mass per volume is insignificant. So much for the mass equation.
When the concentrate and water come together the process they experience is called thermal equilibration. The basic idea is tinitially temperatures are different throughout the water and as time passes a single, uniform temperature is attained all occurring at one atmosphere.
To write the energy equation we will identify three masses. The mass of ice as "icemelt" which melts. Then "icesolid" being that ice at -15°C that is warmed to 0°C but that remains solid and the water that is liquid at room temperature and is cooled to 0°C.
| Normal Propeties of Water | ||
| Specific Heat Solid cp,solid,avg = 1.36 J/(g°C) |
Heat of Fusion hsf = -333.4 J/g |
Specific Heat Liquid cp,liquid,avg = 4.16 J/(g°C) |
The first concept which must be understood in applying thermodynamics is the necessity to begin with the definition of what is called a "system". In thermodynamics this is any region completely enclosed within a well-defined boundary. Everything outside the system is then defined as the surroundings. Although it is possible to speak of the subject matter of thermodynamics in a general sense, the establishment of analytical relationships among heat, work, and thermodynamic properties requires that they be related to a particular system. We must always distinguish clearly between energy changes taking place within a system and energy transferred across the system boundary. We must likewise distinguish between properties of material within a system and properties of its surroundings.