Light Bulb Efficiency

Short-time-heat is radiant only.

As a science project, students decidee to evaluate the efficiency of common 100-watt light bulbs. They defined "bulb efficiency" to be the useful, visible energy produced by the bulb divided by the electric power the bulb expended.

Normal Operation: When a light bulb is turned on, it comes to steady usage of power and steady temperature of operation quite quickly. The tungsten filament becomes incandescent and the glass globe of the bulb becomes hot. To be cool, heat of the glass globe passes convectively from the outer surface of its glass globe to surrounding air. Heat also radiates directly from the filament, through the glass of the globe and into the room. Part of this radiation (heat) our eyes can perceive - the bulb gives light. While some heat is conducted from the bulb up its connecting the wires that power it. Also some radiation of the bulb will be in wavelengths not perceivable by human eye. We assume this heat effects to be negligibly small. The energy equation for a steadily operating bulb is:

(1)

The work term (1) is the 100 watts of electrical power delivered to the filament resistor. Convective heat from the outer surface of the glass bulb, term (2), is not known. Term (3), the radiant energy (heat) that passes from the bulb into the room is also not known. Part of that energy is "useful" being visible as light. Although this energy equation cannot be solved; it is not useless. The equation shows that should a test be devised, wherein the convective heat to air (2) could be measured, then the radiant heat to the room (3) could be determined.

Test Set-Up: The electrical wiring to the bulb is made waterproof and it is submerged in a glass beaker filled with water. Once power is turned on, we expect the filament, stem and glass of the bulb to attain their high, constant, operating temperatures, nearly immediately. The outer glass surface of the bulb will pass heat convectively to the water in the beaker. Heat will radiate (some visible, some not) through the glass and water into the room.

The system will be the bulb, filament, glass globe and water taken together. Once on, the temperature of the water will begin to increase. As the water becomes hotter, heat will commence leaving the outside surface of the beaker by convection to air. However, for the first minute or so, the convective heat from the outer surface to room air (2) will be very small.

The energy equation (a first order differential equation) can be written in terms of enthalpies of its components, the bulb et al, (glass globe, filament and metal base...) and the water (we ignore the beaker).

(2)

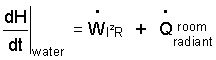
(3)

Prior to the event, power is off and every term of the above equation is identically zero. The equation "comes to life" at "t = 0+" when the power is turned on. In that instant the bulb, et al, become "maximum hot," so dH(t)/dt|_bulb becomes zero. The with water surrounding the bulb blocks convection to air (2). The power become 100 Watts. The energy equation becomes:

(4)

Separating variables we have:

(5)5

The test was conducted for 34 seconds with a temperature increase of the water of 7°C. We use these as limits for the integrations.

(6)6

Recognizing that the radiant light and the power supplied are constant:

(7) 7

We expect the visibly radiant heat to be negative since heat from the system to the surroundings is negative.

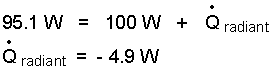
(8)

Our answer is negative meaning energy as heat left the bulb. The efficiency of the 100W bulb in delivering radiant energy to the room is 4.9%. We cannot separate the radiant energy into its visible and not visible parts. However, since some of the radiant energy is not visible, we can state that the efficiency of the bulb as a light source is less than 4.9%.