THERMO Spoken Here! ~ J. Pohl ©

TOC NEXT ~ 1

Rifle Musket (1861)

Lead bullets recovered from Civil War battlefields exhibit severe fragmentation and distortion from their original shapes. Many of these were fired by Springfield rifle muskets. A possible explanation is that impacts of the lead Minie balls caused them to melt partially, smear, then freeze back to solid in a contorted form.
Is it possible the lead bullets actually become liquid upon impact?

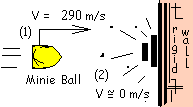
Impact occurs promptly. Hence
heat of the event is zero.

♦ Rifle muskets fired Minie balls with muzzle velocities as great as 290 meters per second. Physically, our question is: assuming upon impact all of the kinetic energy of a ball changes to enthalpy of the lead, will any of the lead have become liquid?

We begin with the energy equation. A sketch of the "incremental" event is shown. A difference form of the energy equation is appropriate.

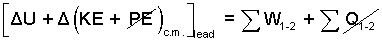
(1) The general form of energy equation
for a mass as system.

Change of potential energy is very small and time span of impact is too short for there to be heat. The lead bullet has two possibilities for work. First, lead (as everything) is a simple compressible substance. Some call this has "pdV" work. Also the lead can do work (as all movies now show) by driving the person being killed (Yankee or Reb) backward yards and yards. Let us study a shot that misses and hits an oak tree.

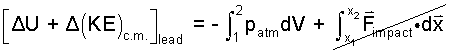
(2)

In the integral, atmospheric pressure is constant. The above integrates as:

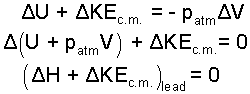
(3) The change of enthalpy and the change of
kinetic energy add to equal zero.

Enthalpy change, ΔH, equal mass of lead times its specific enthalpy change, mΔh, where Δh = (h₂ - h ₁)_lead. We assume the second kinetic energy after impact to be zero.

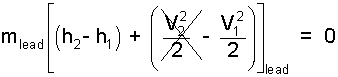
(4) 4

Eqn-4 has the algebraic form, "A x B = 0," wherein either A or B must equal zero. It is absurd to set the mass of the lead equal to zero. The specific energy of velocity, as a side calculation is 42J/g. Eqn-4 becomes Eqn-5.

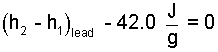
(5) 5

The above single equation contains essential but insufficient information to establish whether in its second state, any of the lead might be liquid. We need one more valid equation.

LEAD at 1 atmosphere
SOLID	MELT/FREEZE
ρ_s = 11.3 g/cm³ c_s= 0.13 J/g°C	T_melt = 327°C h_sf = 23 J/g

Property relationships expressed as an equation, graph or table constitute an "equation." Specifically we need values of enthalpy for lead.

Data we can use belong to the set: "Normal Properties of Lead." A search of the internet yielded the following information in its typical format. Table in hand, we recast Equation (5) as:

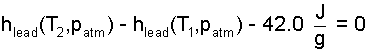
(6) 6

One might think the table would provide a specific value of enthalpy of lead, say h_lead,1 = h(25°C, 1 atm). But we find the value not listed. Normal Data do not provide enthalpies specifically. Rather, the data are a means of constructing "enthalpy differences." The table contains temperatures of phase change for melting/freezing and average values for specific heats for the phases. The manner of using these data is to construct the "enthalpy difference," as shown below.

Since enthalpy is synonymous with energy (and temperature), we anticipate the second temperature of the lead to be greater than its first temperature. We further assume the second state of the lead to be all solid. To express the "difference" we write (on a first line) the second enthaply (h₂) in terms of the first (h₁). Then on a line below we write the first enthalpy (h₁).

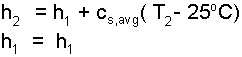
(7) The change of enthalpy is expressed as its
average specific heat times its temperature change.

Next, in Eqn-7, subtract the lower equation from the upper which yields the difference, Eqn-8:

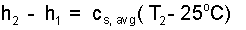
(8) 8

Here, c_s,avg represents the average specific heat of solid lead on the temperature range 25°C to T₂. Since we don't know T₂, we can only approximate c_s,avg. We take the average value to be the table value; 0.13 J/g°C. (Note: we can later refine this approximation). We substitute Eqn-8 into Eqn-5 and solve for the second temperature.

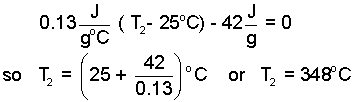
(9) The use of an approximate average specific heat allows
us to proceed to a solution; to obtain an approximate second
temperature, from which (availability of data permitting) a
second, better average specific heat can be determined, and
with which a second iteration of calculation can be made...
The process will converge.

So what do we have? To expedite solution we assumed the final state would be solid. We cast the second enthalpy from that perspective. Carrying that assumption our solution is that the second temperature of the lead is 348°C.

However property data indicate lead is LIQUID for temperatures greater than 327°C. We have a contradiction. Since our answer is wrong, our assumption was wrong. Nonetheless, that calculation has value. "All solid" is excluded from reality. There will be "some solid and some liquid" or there might be all liquid or... with patience we will learn what happened to the Minie ball.

Second Calculation: Next, we assume the second state of the lead will be both solid and liquid phases. When a substance exists as solid and as liquid (in equilibrium... same T and p) the solid is called (historically) a "saturated solid" and the liquid a "saturated liquid." Both phases have the same temperature and pressure but the enthalpy of the liquid phase is greater than that of the solid phase by the amount of the "laent heat.".

Higher energy of the second state is expected. We must recast h₂ - h₁. The steps begin with the energy equation which is repeated, Eqn-4:

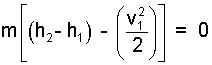
(10) 10

We arrange the equation to have mh₂ alone as a term.

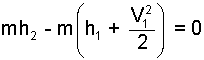
(11) 11

=============================
Now, separate from Eqn-11, we are going to rearrange mh₂ to reflect that the lead after impact is part solid (s,2) and part liquid (f,2) ("f," means fluid or liquid). Finally the mass is:

(12) 13

The second enthalpy, H₂ (or mh₂) will equal the mass of solid times the saturated solid enthalpy added to the mass of liquid times the saturated liquid enthalpy, Eqn-13.

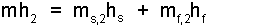
(13) 13

The right side is multiplied and divided by the mass (sum of components).

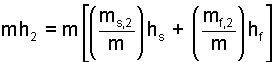
(14) 14

But we also have:

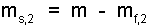
(15) 15

Upon substitution of Eqn-15 into Eqn-15 and rearranging (just a tad) begets Eqn-16.

(16) 16

Here it is appropriate to introduce two definitions. Of the phases, solid and liquid, liquid has the higher energy. For any two-phase situation we define quality, x: to be the ratio, "mass of the higher energy component" divided by "the sum of masses (both components)" - Eqn-17.

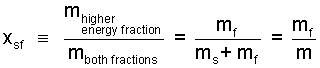
(17) 17

The latent heat of fusion (same as melting) is the energy difference: enthalpy of saturated fluid minus enthalpy of the saturated solid.

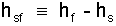
(18) 18

Now insert the definitions, Eqn-17, 18, into the result of Eqn-16 yields Eqn-18commonly used definitions permit mh₂ to be written as:

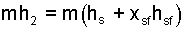
(19) 19

This result is used to replace mh₂ in our energy equation, was Eqn-10, is now Eqn-19.
==========================================

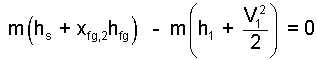
(20) 20

That system mass divides from this equation means the same result would apply however small or large the lead projectile.

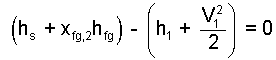
(21) 21

Since the event is a difference type, we regroup the equation

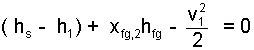
(22) 22

This result merits a moment of discussion. Our previous calculation indicated a final specific energy of the lead that was higher than "all solid" would permit. In the equation, the difference ( h_s - h₁) is positive. It represents the energy increase all of the lead experienced as its temperature increased from 25°C to 327°C. Quality, x_sf, is positive as is the latent heat, h_sf. Their term represents the energy increase of the fraction of the lead, initially solid, that became liquid. And the kinetic energy change is negative. We can discuss there terms because we have left then "where they were." Just a little algebra can destroy such possibilities.

The enthalpy difference (h₂ - h₁)is expressed as an average specific heat times the melting temperature minus initial temperature. The latent heat (from Lead @ 1atm) ~ 23 J/g is entered. The initial specific kinetic energy (as calculated before) is returned to the equation (42J/g).

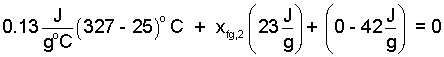
(23) 23

Solution of Eqn-22 yields the second state quality as 0.12. Quality is positive with two phase liquid/solid. The number we obtained is positive - hence the assumption is proven, after use of the fact.

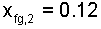
(24) 24

Assumptions of our analysis were not severe. We conclude a solid lead Minie ball fired from a Springfield rifle musket upto impact with something totally solid would experience a 12% transformation to liquid.