THERMO Spoken Here! ~ J. Pohl ©

TOC NEXT ~ 185

Cyclist Power

Simply stated, a bicycle race is a competition in which each cyclist "expends physical energy to move him/herself and the cycle along a road through the surrounding air." In places the road is flat and further along a hill must be climbed and once at higher elevation, a descent is required.

Calculate the steady power expended by the rider during a time trial, a climb and a downhill.

Steady Cycling at Constant Speed: The general topic of cycling is too broad a task for us. To narrow the topic we will focus on the steady, constant speed, parts of the stages. For the majority of a race the rider and cycle will move essentially at constant speed. Of course short bursts of effort will occur during which speed is changed but for a great part of the time as during a time trial, as during a steady climb or during a downhill, the cycle and rider move together at near constant speed. We will analyze these "constant speed" aspects.

As numbers for analysis use the following:
i) The rider mass is 70 kg, the mass of the cycle is 6 kg.
ii) The time trial was 6.1km, traveled in 6 min 52 sec. Average speed - 14 m/s.
iii) The climb considered has a 7 meter rise in 100 meters (horizontal).
iv) The downhill slope is down 10 meters per 100 meters horizontal.
v) Drag data: projected area ~ 0.6 m² Air density ~ 1.2kg/m³ coefficient ~ 0.88.

♦ The first step of an analysis is to choose the subject to be studied, identify that mass that has an event ~ the system. Clearly, "cycle and rider" is the system of racing events. This choice is uncommon in that it is a combination of an animate part and an inanimate part. System as "cycle and rider" is appropriate but we will develope our system equation by consideration first of "cycle as system," next of "rider as system" and finally "cycle plus rider as system" being the sum of the "cycle perspective" and the "rider perspective."

CYCLE as system: Eqn-1 is the rate-form-energy-equation. Constant speed means constant kinetic energy. Thus ~~dKE/dt~~ is set equal to zero.

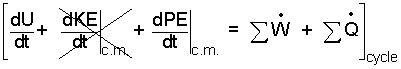(1)

Energy Equation: CYCLE

To expand the summation of works we realize work occurs to the cycle in two general ways. Cycle energy is increased by the forces of the rider applied to the pedal tops through their downward displacements. Also, motion of the structuree through air is opposed by that air - this is called aerodynamic drag. (There is also rolling friction. As a simplification, we assume rolling friction to be negligible).

(2)

Energy Equation: CYCLE

Terms (i) and (ii): Internal energies of the light-weight cycle materials are functions of specific volume, and temperature, i.e., u = u(v,T). Material specific volumes are expected to change inconsequentially during a race. Thus, term (i) relates to temperature changes of the cycle. Initially at rest (with no rider) the cycle will have a steady, distributed temperature, no change of PE and no manners of work. Eqn-2 becomes Eqn-3(left):

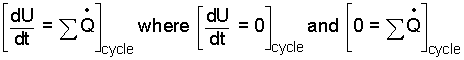
(3)

Eqn-3... expanded.

Eqn-3 is expanded. dU/dt is zero because temperatures at cycle material locations are constant. Sum of Q-dot is zero but virtue of the sum. Heat of the cycle part in the shade summing with that in the sun. Individual heats are not zero - their sum is.

Rider mounted, cycle in motion, a new equilibrium will happen. Some describe this as having a mechanical and thermal transients. Internal, solid-on-solid relative-movements of parts constitute internal friction resulting in localized temperature increases (at the expense of pedal-power). Heat might occur to the cycle at places of rider body contact. Regarding operational thermal changes, Eqn-3 shows the likely, steady equality. Hence we assume Eqn-3 to apply to our analysis of each stage.

Term (iii): Drag is a system work interaction. The surrounding air opposes motion of the cycle through it. The force and velocity of its dicplavement are opposite. Thus the work is negative and for stesdy motion of the cycle, this work represents a steady decrease of system internal energy. It represents a A drag force acts on the cycle boundary. The force opposes cycle motion. It appears right-of-equality in our energy equation.

In Eqn-2, the work of drag shows a special notation. To write "-|W-dot|_drag" is to mean the term is always negative. Again, left-of-equality are energy rates of the system, the effect of friction is to remove energy from the system - hence the negative sign. Removing Eqn-3 from Eqn-2 we have:

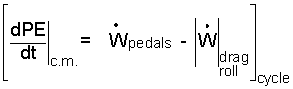
(4)

Cycle is part of the relevant system. Rider is the other part.

=====================

Rider as system: The energy/work/heat interactions of the human rider are poorly represented by physics. We use physics anyway. We assume the "sum of heats" to be inconsequentially small. An approximate energy equation of rider as system moving at constant speed is:

(5) (5)

(6) (6)

Eqn-6 is our conclusion - Rider as system.

Cycle and Rider as system: The equation we need includes the terms of Eqn-4 and Eqn-6 with a slight change. When the cycle boundary and rider boundary are combined there is a section they have in common. This is the tops of the pedals. When the boundaries are combined the section at the pedals becomes "interior." When the cycle system and and rider system are joined, the work interaction of the rider and that of the cycle cease to happen. There is energy change at the pedal still but it is not called work; it is called - mechanical equilibration.

(7) (7)

Next we represent the work of drag as the drag force times velocity of the cycle/rider system.

(8) (8)

Racing teams have studied drag extensively. The resisting force always opposes motion. Its magnitude is directly proportional to the area of a "frontal" projection of cycle and rider, A_f. It is directly proportional to the air density and to the square of the relative speed. (A factor of 1/2 is used to have a pattern with kinetic energy). Usually expensive experimentation is required to determine the drag coefficient, "C_D."

(9) 9

Drag force opposes motion in proportion to the square of the speed. The associated work is negative, meaning energy must leave the system.

(10) 10

Bicycle racing is highly competitive; plenty of money is spent preparing riders and testing equipment. Approximate numbers for the drag coefficient for all aspects of performance are determined for each rider/cycle-combination. The frontal area of this investigation is 0.6m², the density of air as 1.2kg/m³ and the drag coefficient, C_drag equals 0.88. (For the sake of simplicity, We will use only one number for drag coefficient). This information entered into the equation yields a result suitable for any phase of a race.(all_phases)

(11) 11

Eqn-11 is our general equation. It applies to the three cases.

Time Trial Power The rider completes a ride of 6.1 kilometers in 6 minutes and 52 seconds or an average speed of 14 meters per second. Calculate the steady watts of physical energy expended during the race. Since the time trial events occur on horizontal roads, potential energy changes equal zero.

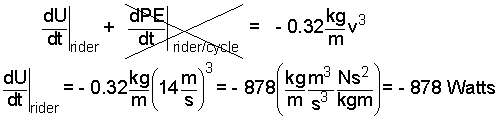
(12) 12

The power is negative meaning the energy of the rider decreases.

Constant Speed of Climb: During climb, the rider/cycle system will experience a steady rate of potential energy change equal to the mass times gravity times the vertical component of the road speed.

An intermediate calculation is needed:

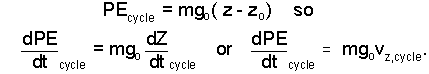
(13) 13

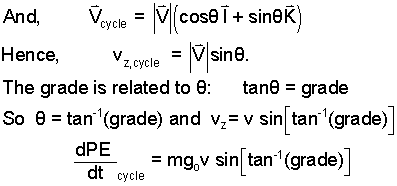
(14) e14

Now return to the climb. We assume the rider capable of delivering the same sustained power in climbing as for the time trial. The grade is 7 in 100. Eqn-14 defined that power need. Drag (right-of-equality) changes as the speed cubed.

i05

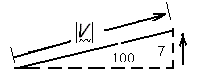
(15) e13a1

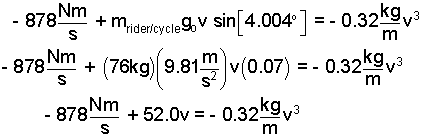
(16) e13a2

A factor of the rate of potential energy change of the rider and Potential energy of the rider and cycle are related to road speed. The uphill grade is 7%. We Our energy equation we realize rate of climb is part of road speed. We can write dPE/dt in terms of the cycle speed and the grade of the slope.

We will apply this energy equation for the constant speed events of time trial, downhill coast, and climb.

Constant Speed Downhill: The grade at 10% represents a steep, dangerous downhill. Our cyclist (no madman) will rest and coast.

i05

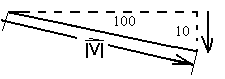
(17) e17

About assumptions: Though we dislike assumptions, the task of engineering analysis is to arrive at some answer. If, while analyzing things that might happen, one arrives in a mental corner, assumption can be a substitute for despair. Reasonable assumptions are preferred, of course. Necessary assumptions distort the consideration to some other situation; what is the new situation. When progress seems impossible. If an absurd assumption comes to mind and is applied to the model of physical reality - mathematical nonsense should result.