| THERMO Spoken Here! ~ J. Pohl © | TOC NEXT ~ 138 |
3.06 Forklift Events
The previous derivation of the energy equation for a BODY (sometimes called the "extrinsic energy equation") was accomplished mathematically without reference to anything specifically physical. Below we will derive the same equation but do so in reference to the physical events of a Barrier Section (BS) as it is moved by a forklift.

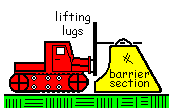
A forklift pushes and lifts as it arranges sections of vehicle-barrier about the perimeter of a military compound. (The barrier sections will be notated as BS .) The momentum equation governing the motion of a BS and a component form of its velocity (in an 0XZ coordinate space) are written:

| (1) Newton's Momentum Equation and velocity in component form. |
Pushing Event: As one concrete section was forced to slide across gravel-covered ground (assume no sliding friction), it impacted a small tree stump and stopped. The sketch (right) shows the forklift ( FL ), barrier section ( BS ) and stump configuration.
The system model we will use is "extended body." The system free-body-diagram (below right) shows four forces, all directed through the center of mass of the system. The momentum equation with the section as system is written below left.

 |
(2) 2 |
Neither the force of the stump, Fstump, nor the horizontal pushing force of the forklift, FFL, has a force component in the upward or K direction. Thus the gravity force and normal force of support by the roadway are equal and constant. The vertical component of the sum of forces being zero means that left-of-equality, d(m v z)/dt = 0.
The remaining, non-zero, I - component terms of the momentum equation and an I - component of BS displacement are written:

| (3) 3 |
Physically, the BS and forklift are stopped. Though being pushed, the momentum term of the BS (term left of equality) equals zero and the sum of forces (right of equality) also equals zero. "Objects at rest remain at rest until ΣF becomes non-zero," or unbalanced, as some say.
To get on with the job, the forklift driver "powers-up" to apply a slowly increasing force to the block which transmits through it to the stump. Imagine the resistance force of the stump to increase slowly. Briefly, the stump resists. The difference, 0 = |F| FL,x - |F| stump, remains zero.
When the stump fails, motion will ensue. But before that, let's take a line or two to alter the momentum equation. Our task is to set it by vector multiplication to be applicable to a scalar, differential (x-direction) displacement.

| (4)
♦ The entire vector differential equation is scalar multiplied an x-component, vector differential displacement. ♦ The multiplication is applied to the terms individually. ♦ In each term “I dot I” equals 1. ♦ A scalar differential equation results. |
The time in our discussion is still "prior to event," t < 0+ . The right side of the above equation is zero, therefore so also is the left side. Nothing is happening! But the force applied by the forklift is increasing. When that force exceeds the capacity of the stump to resist, (at the time, t = 0+), the stump shears (assume instantly) whereupon the stump force, |F| stump, becomes zero. But |F| FL,x is at a "powered-up" (not zero) value, the event initiates, the BS moves.

| (5) 5 |
This equation is in differential form. Some changes of notation can be applied:

| (6) 6 |
Assure to yourself that these steps are correct.

| (7) 7 |
The symbol for kinetic energy, KE x, is substituted into the equation (above left):

| (8) 8 |
Care is being taken to represent horizontal pushing of the barrier as an "X" component. We leave this result to return momentarily.
LIFTING EVENT: Next the operator inserts the forks of the lift into the lifting cable of a barrier section and begins to lift, slowly. To the right is a configuration sketch. Below right is a free body diagram of the barrier section modeled as an extended body.

Again we use Newton's Momentum Equation with velocity written in component form.
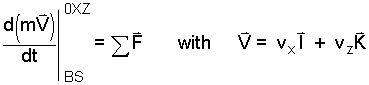
| (9) 9 |
The forces are identified:

| (10) 10 |
Briefly, as the lifting equipment pulls snug, three forces act with the barrier section velocity remaining zero, vz,BS = 0 .

| (11) 11 |
As the forklift takes the load, the normal (supporting) force vanishes. Millimeters higher, the barrier section starts up at constant speed (uniform vertical motion). The differential momentum equation has a left-of-equality term equal to zero and therefore the sum of forces also equals zero. As before we need a differential displacement. These are written as:

| (12) 12 |
By the same math procedure as "pushing event," scalar multiply the momentum equation by the differential displacement (steps omitted).

| (13) 13 |
The force of the forklift is identically equal to the gravity force acting on the mass as the mass moves upward uniformly at constant, but not zero, momentum.

| (14) 14 |
Were the force of the lift increased to become greater than the gravity force, that is if |F| FL,z became the larger force (notated with a plus, + , |F| +, a change of vertical momentum, an increase in upward velocity of the barrier section would occur. The equation form expressing this is:
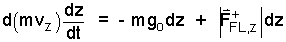
| (15)15 |
Simplifications similar to before yield:

| (16)16 |
The first term right-of-equality is "work against gravity." This term is quite simple and also outwardly observable. The approach of thermodynamics is to move this term from the right, work or energy transfer side of the energy equation to left-of-equality or the "system possesses energy side" of the equation and to rename it: potential energy. In effect this changes our system from being the barrier section to being the barrier section and earth. Note in this development, that "go," a factor of potential energy, belongs to Earth. Also since elevation, Z, is zero at the surface of earth, the surface of Earth is a datum for zero potential energy.

| (17)17 |
Combined Event : Now, while the case of "pushing" considered above was in the 0XZ plane, it makes sense that to write the result for an event in the 0YZ plane we need only change all letters corresponding to "X" to "Y." Thus "pushing" in 0XZ and 0YZ are written below,respectively, with the "lifting result" beneath them.

| (18)18 |
and for "lifting...

| (19) 19 |
Since physically it would be near impossible to move the barrier section ( BS ) without a little bit of X and Y and Z action, these mathematical equation pieces which purport to describe reality, must have a sum. Indeed, the energy terms add nicely and immediately. To add the components of force times their displacements we return them to their scalar product form:

| (20) 20 |
In putting the equation pieces together, we use ΣF since we are headed toward generality.

| (21) 21 |
We used a section of vehicle barrier in this development. Obviously the results can be applied in general. For the event of any arbitrary (non-rotating) body we have:

| (22) 22 |
Consistency of plan and action is a good idea in thermodynamics. A way to proceed should not be abandoned until defeated by a better one. So, to return the the path of Newton's Second Law, lets express our result as a first order differential equation with time as the independent variable. The step requires simply to divide by "dt."

| (23) 23 |
Velocity is recognized:

| (24) 24 |
The final form is:

| (25) 25 |
The compounded, subscripted notation, c.m. (for center of mass) and BODY mean "extended BODY" or "BODY."

Forklift Events
The previous derivation of the energy equation for a BODY (sometimes called the "extrinsic energy equation") was accomplished mathematically without reference to anything specifically physical. Below we will derive the same equation but do so in reference to the physical events of a forklift.
Premise presently unwritted!