| THERMO Spoken Here! ~ J. Pohl © | TOC NEXT ~ 32 |
1.13 Measurement of μMEarth
Newton (and his colleagues) postulated that all masses experience mutual and equal attractive forces with other masses as a consequence of their existences. Such masses of the universe include the sun, planets, stars and so. With common, surface-of-Earth scenarios only gravity of Earth is significant. Here, for simplicity, we address gravity consequences of Earth with a small steel sphere.
If both the ball and Earth are taken together, as a system, gravity forces are interenal and sum to zero. However we take the ball as system (separated, so Earth becomes part of the surroundings). From this perspective the ball will experience a gravity force in a way it can be made quantitative.
Newton's (and others) quanlitativly postulated gravity by the equation: Eqn-1.

| (1) 1 |
For the steel ball system, of the equation terms, mass was known quantitatively as well as the radius of Earth (measured by Eratosthanes). Further, since most usages of the equation would be at the Earth surface, the radius, r, could be set equal to ro,Earth. After these considerations two unknowns of the equation remain: the proportionality constant and the mass of Earth; neither known quantitatively. To proceed to express gravity force quantitatively, Newton and others were obliged to combine the last two unknowns to be a product, μME - to be dermined by experimentation.

The sketch of an experimental apparatus is shown to the right. An iron sphere is placed inside a tall, air-tight glass cylinder and held against the cylinder top by a magnet. Much of the air is removed from the cylinder by an air pump. The apparatus is located on Earth (z = 0 and r = ro) which is assumed to be an inertial reference with velocity equal to zero. In the sketch of the apparatus no forces are shown. Forces are constructs that determine the equilibrium of the sphere once it is extracted from the universe to become a system. Forces arrive upon isolation.
In the next sketch (to the right), the iron sphere is shown as our system. We choose to model the physical sphere as a distributed mass of iron. Forces of gravity and magnetic attraction that act on the sphere are indicated as being distributed.

There are also forces of iron-to-glass contact that act over parts of the surface of the sphere. These forces are depicted in this sketch (as best as possible) as they actually are - distributed.
Distributed forces are tedious to draw; engineers usually bypass that detail by representing the forces immediately as their single, "summed-effect" equivalences. The single force arrow results as a "sum of distributed force, located by a sum of moments of the distribution.
The effect distributed gravity forces becomes a single gravity force acting through the center of mass of the sphere. The magnetic and contact forces are likewise summed and represented as single forces acting through their respective centers of force. Next coordinate axes and a vector basis are added to describe the 0XZ space. The reduced system is shown below:
Newton's Law of Accelerated Motion will be applied to the constrained sphere for all times prior to the event.

| (2) 2 |
In the physical state being discussed, application of the law yields two consequences: the momentum of the sphere is constant and the sum of forces acting on the sphere equals zero. Nothing is happening, the condition is the state of static equilibrium or "permanence."

Time is expressed here as "time less than zero-positive, t < 0+" which is a way of saying all times prior to the event.

| (3)
Before the Event: The velocity equals zero and the “sum of forces” equals zero. |
By the physical nature of the system an event is anticipated. The magnet can act as a "trigger" or catalyst. The instant the magnet is snatched away, our terminology will be to call that instant of start: time equal to zero-positive, written as, t = 0+.

The sketch (right) shows our steel sphere the instant the magnet is snatched away. Both the contact force and magnet force have vanish, leaving only the gravity force. The event that ensues is called free fall. This is an event in which momentum changes and the sum of forces is not zero.
Newton's Law of Acceleration, a vector differential equation, is a single statement, a governing principle of motion of a body in general. To apply the equation specifically, as in the case of this "sphere just falling," appropriate forces and initial conditions are specified. The equation and conditions are:
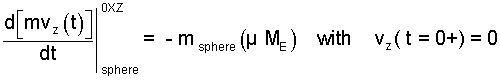
| (4)Differential equation with its initial condition. At t = 0+." |
Integration of a first order differential equation follows the steps: separate variables, apply integration and place appropriate limits:

| (5) Separate variables and integrate. |
Represent v(t) as dz(t)/dt then separate variables and integrate again.

| (6) Integrate once again..." |
Thus, had Newton been able to accurately measure the distance and time of free fall of a body, he could have determined the value of μ MEarth. But that was difficult because a fall of 10 meters (~30 feet) requires only 1.4 seconds (as we now know). Clocks in the 1650's were inaccurate at timing such events. Ways other than direct free fall had to be found. Two such alternative ways discussed here are Galileo's Inclined Plane (shortly below) and Atwood's Machine.
The magnitude of μME has been determined to be (of sufficient accuracy for our purposes): 9.81 m/s² (or 32.2 ft/s²). The group μMEhas been given a new symbol, go,E which is called the "Earth surface acceleration of gravity." When this result is substituted into Newton's postulated equation the magnitude of the force of gravity is made quantitative as:

| (7) 7 |
Equation (7) shows the dependence of the force of gravity on "r", the distance from Earth's center to the BODY. For many engineering calculations the approximation, r ~ ro is reasonable such that, |Fgravity| ~ msysgo| .
1.14 Measurement of μMEarth
Newton postulated that all masses experience forces as a consequence of the Earth mass. He called this phenomenon, gravity. Gravity forces which act on all parts of mass of a system are directed toward Earth. The magnitude of the Earth gravity force was postulated by the equation. The challenge was to make this force measurable or quantitative.
Premise presently unwritted!