Train Passes Two Boys

Two boys, walking beside railroad tracks heard a train approaching from behind. Their backs to the train, they continued to walk. The older boy knew city train speeds were limited to 30 mph (44 feet per second) and that he and his buddy walk about 3 feet per second. When the nose of the engine was abreast of them, the smaller boy (his job) began to count. Later, the end of the train (to which a small flag is attached) passed. The count, at the instant the flag was beside the boys was, ".. 33, ... 34 seconds." In a moment the older boy said, "A short one, only 1400 or so feet long."

Was the kid's estimate about right?
♦ The older boy used the count of seconds while he walked at a steady pace parallel to the moving train. We will explain his thinking, how he estimated the length of the train by use of vectors. While plain algebra would suffice, our goal is the method of vectors. First we consider the extremes of this event by sketching them.

Event State upon Initiation: The sketch depicts the boys (moving with speed, 3 ft/s) with the train immediately abreast and moving past them. Its speed is 44 ft/s. This instant shown is the starting time of our event.

We associated the time (at that instant) as "commencement" of the "train-length measuring event." We notate that time as "time equal to zero-positive, "t = 0⁺." The meaning of "zero-positive" is the idea "a clock has been started and is running." At that instant (clock running) we imagine establishment of a one-dimensional coordinate reference (an X-axis) with its increasing direction toward the destiny of the train. We place the origin or zero value of that axis at that instant on the ground beneath the boys. As vector basis we select the unit vector, I, directed to coincide with positive X.

Event State upon Termination: The boys mark "end of the event" as that instant We discuss the termination of this event next. The sketch shows characteristics of the event at its moment of termination, a time we call: t = 0⁺ + 34 seconds."

Event States Intermediate: Mathematically speaking; vectors span space. We will use one-dimensional vectors on this simple "straight-line-motion" problem. We set an origin to be a point on the solid, non-moving ground directly beneath the boys at the instant counting commenced.

For our vector basis we need only one unit vector; we name the direction the train advances on the tracks to be the positive X with the unit vector, I direction.

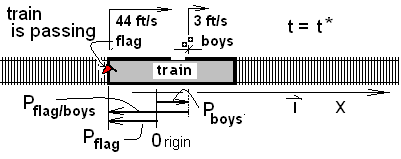The physics here involves three connected vectors as they change in length over the passage of time of 34 seconds. These vectors are the position of the boys, the position of the end of the train (where the flag flies) and a vector that extends from the boys to that flag.

The sketch shows the three vectors at the initiation of the event, of counting,... at the time we call t = 0⁺.

The position of the boys (at the origin) is P_boysI. The sketch (right) shows three vectors:

We define an 0 - X one dimensional vector space as an x-axis parallel to the tracks. The unit vector we need is I. A one dimensional vector equation that describes our system of points is:

P_B(t) + P_F/B(t) = F(t)

(1)1

The sketch shows the flag and boy at an arbitrary time of tie event, t*, where 0+ < t* < 34s .

Taken individually, the boys and the train are in uniform motion. Together they constitute a relative uniform motion event. The position of body in uniform motion is written:

P_body(t) = P_body,0+ + V_bodyt for all 0+ < t.

(2)2

We specialize the above expression for the boys (B) and for the flag (F) on the end of the train as:

P_B(t) = P_B,0+ + V_B t for all 0+ < t

(3)3

P_F(t) = P_F,0+ + V_F t for all 0+ < t

(4)4

Next we substitute these expressions into our first vector equation.

P_B,0+ + V_B t + P_F/B(t) = P_F,0+ + V_F t

(5)5

These terms are vectors in the direction,I. At time,t = 0+, the boys are at the origin (0 I) and the position of the flag is L(- I). Their speeds are 3ft/s I and 44ft/s I respectively. Thus our vector equation becomes:

0 I + (3 ft/s I) t + P_F/B(t) = L (-I) + (44 ft/s I) t

(6)6

Physically, the event ends when the flag is opposite of the walking and counting boy. At that time the vector position of the flag relative to the boy, (P_F/B) has zero length Notice its direction is not zero; its magnitude is zero). We rearrange to have P_F/B, left of equality.

P_F/B(t) = - L I + (44 ft/s) I t

(7)7

This equation fits the initial condition. At time, t = 0+, the flag is L feet behind the boys.

Now at t = 34 seconds, the boy and flag are beside each other. Hence:

P_F/B(t = 34s) = 0

(8)8

0 = - L + (44 ft/s)( 34 sec) and, L = 1394 feet.

(9)9

Persons good at high school physics might scoff at this solution saying, "This problem is easy. Why do you work so hard?" Yes, the problem was easy. Furthermore the answer is useless. The problem was posed and solved to show the power of the vector approach at a simple level.